Here we will learn about making x the subject of an equation or a formula.
There are also rearranging equations worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
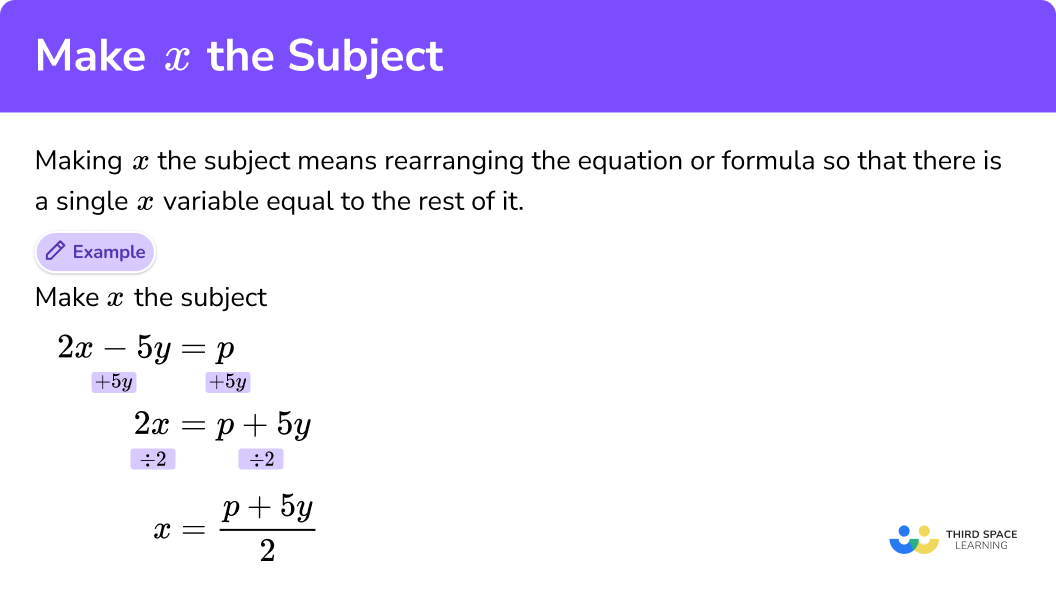
Making x the subject of a formula or equation means rearranging the equation or formula so that we have a single x variable equal to the rest of it.
Make x the subject.

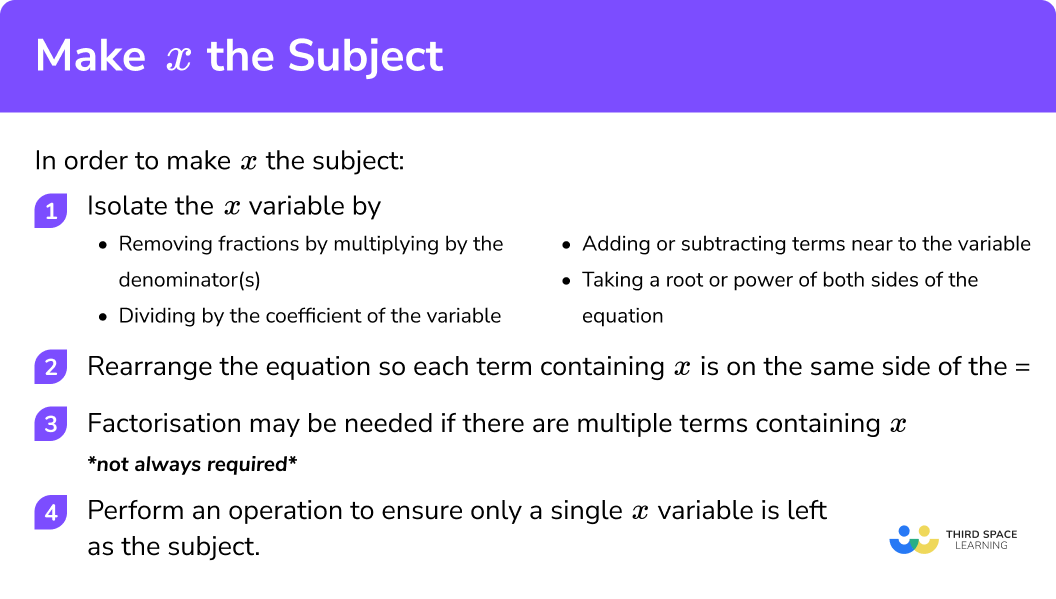
In order to make x the subject:
E.g. factorise 2x + 3xy to x(2+3y)
*not always required*

Get your free make x the subject worksheet of 20+ questions and answers. Includes reasoning and applied questions.


Get your free make x the subject worksheet of 20+ questions and answers. Includes reasoning and applied questions.
Make x the subject.
Step 1:
Divide each side of the equation by 3
Step 2:
Subtract a from each side of the equation
Step 1:
Subtract t from both sides of the equation
Step 2:
Square root each side
Remember the square root can be + or \;−
Step 1:
Add 9a to both sides of the equation
Step 2:
The inverse operation of ‘square root’ is to ‘square’ each side
Step 3:
The inverse operation of multiply is divide, so divide both sides by 5
Step 1:
Multiply each side of the equation by the denominator
Step 2:
Expand the bracket on the left hand side of the equation and rearrange the equation. This will help us to get all terms with x onto one side of the equation
Step 3:
Factorise the left side of the equation so we have a single variable x.
Step 4: Divide by (a - 5b)
This will leave x as the subject of the equation
Step 1:
Multiply each side of the equation by the denominator of the other side.
Step 2:
Expand the bracket on the LHS and RHS of the equation and rearrange. This will help to get all terms with x onto one side of the equation
Step 3:
Factorise the left side of the equation so that we are left with only one of the variable x.
Step 4:
Divide both sides by (b + 8) to leave x as the subject
When we perform an operation to the left hand side of the equation we have to perform the same operation to the right hand side.
To isolate the variable x we need to multiply both sides by 2.
This is wrong because we have only multiplied the 3y by 2.
The correct answer should be:
This is correct because we have multiplied everything by 2 using brackets.
E.g.
To isolate the variable x from 5x we need to perform the inverse operation.
5x = 5 × x , so the inverse operation is divide by 5 .
\[\fracso the inverse operation is × 5.
E.g.
To isolate the variable x from x+5 we need to perform the inverse operation:
The inverse operation of +5 is −5.
E.g.
To isolate the variable x from x−5 we need to perform the inverse operation:
The inverse operation of −5 is +5.
To make x the subject there can only be one x visible at the end. We need to get all x ’s on one side of the equal sign and then factorise.
\[\beginWhen we square rooting a number/variable as an inverse operation the answer can be positive or negative.
\begin & ^>=4 \\ & x=\pm \sqrt=\pm 2 \\ \end \[\sqrt1.Make x the subject of the formula.




Divide both sides by 6
Then subtract 8 from both sides
2.Make x the subject of the formula.




Add 4b to both sides
Square root both sides
3. Make x the subject of the formula.




Square both sides
Add 8 to both sides
Divide both sides by 7
4.Make x the subject of the formula.




Multiply both sides by 5x
Subtract 4x from both sides
Factorise the left hand side
Divide both sides by the quantity in the bracket
5. Make x the subject of the formula.




Multiply each side of the equation by the denominator of the other side.
To both sides, add 6x and subtract 3y
Factorise the left hand side
Divide by the quantity in the bracket
1. Make x the subject of the formula
Show answer(1)
(1)
2. Make x the subject of the formula
Show answer(1)
(1)
3. Make x the subject of the formula
Show answer(1)
(1)
(1)
(1)
You have now learned how to:
Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

© 2024 Third Space Learning. All rights reserved.
Third Space Learning is the trading name of Virtual Class Ltd
We use essential and non-essential cookies to improve the experience on our website. Please read our Cookies Policy for information on how we use cookies and how to manage or change your cookie settings.Accept
Privacy & Cookies PolicyThis website uses cookies to improve your experience while you navigate through the website. Out of these, the cookies that are categorized as necessary are stored on your browser as they are essential for the working of basic functionalities of the website. We also use third-party cookies that help us analyze and understand how you use this website. These cookies will be stored in your browser only with your consent. You also have the option to opt-out of these cookies. But opting out of some of these cookies may affect your browsing experience.
Always EnabledNecessary cookies are absolutely essential for the website to function properly. This category only includes cookies that ensures basic functionalities and security features of the website. These cookies do not store any personal information.
Non-necessaryAny cookies that may not be particularly necessary for the website to function and is used specifically to collect user personal data via analytics, ads, other embedded contents are termed as non-necessary cookies. It is mandatory to procure user consent prior to running these cookies on your website.